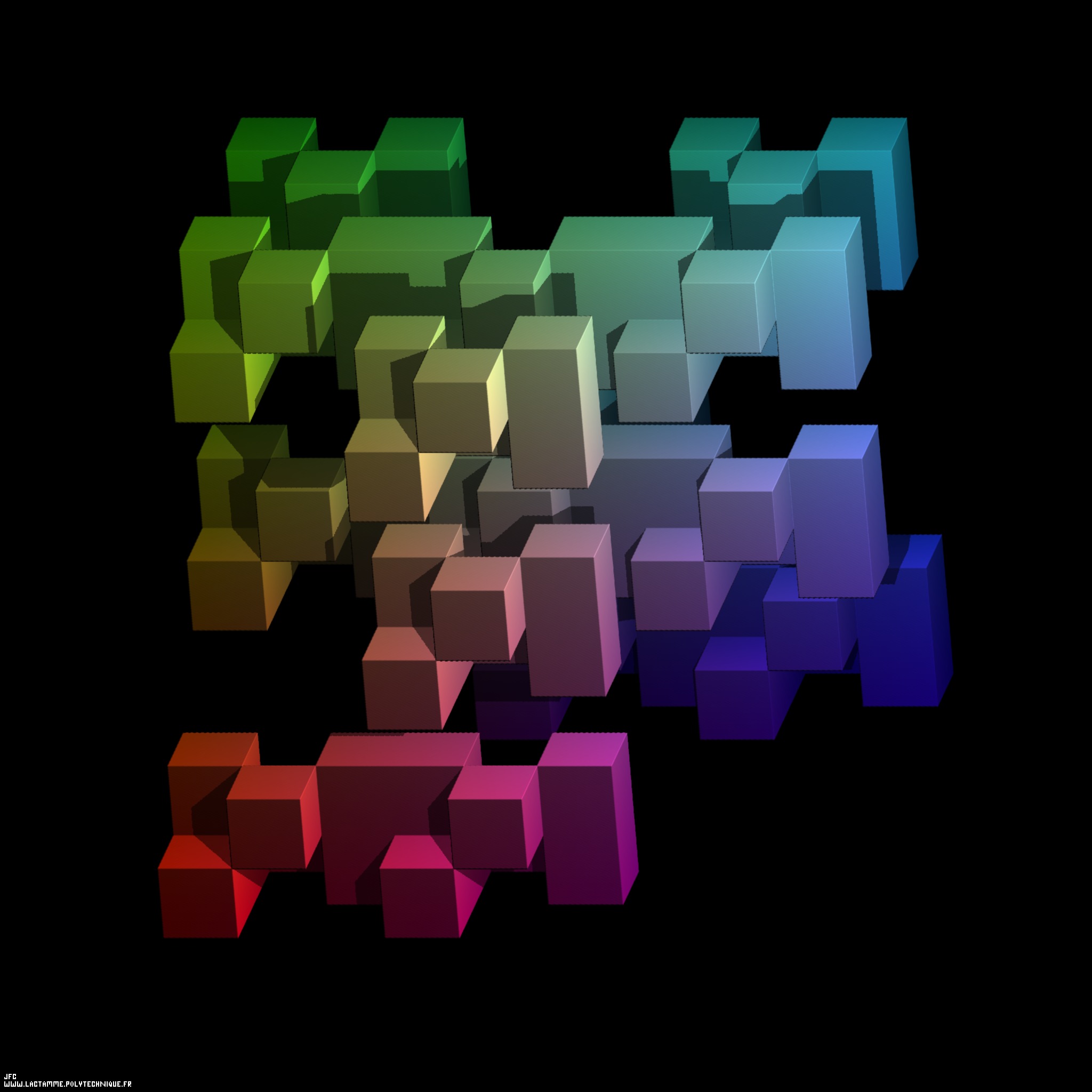
An extended Menger Sponge -iteration 2- displaying the 54 first digits -base 2- of 'pi' [Une éponge de Menger généralisée -itération 2- visualisant les 54 premiers chiffres -base 2- de 'pi'].

An extended Menger Sponge -iteration 2- displaying the 54 first digits -base 2- of 'pi' [Une éponge de Menger généralisée -itération 2- visualisant les 54 premiers chiffres -base 2- de 'pi'].
log(20)
--------- = 2.726833027860842...
log(3)
The "standard" Menger sponge can be defined by means of subdivision rules.
Here is the way how each of the 27 cubes of the "standard" Menger sponge at a given level is subdivided:
"standard" Menger sponge
_____________________
/ \
TTT TFT TTT
TFT FFF TFT
TTT TFT TTT
\_/
Sierpinski carpet
or again:
TTT TFT TTT TFT FFF TFT TTT TFT TTTwhere 'T' ('True') and 'F' ('False') means respectively "subdivide the current cube" and "do not subdivide and destroy the current cube". The rules are repeated at each level, but they can be changed periodically and for example:
TTT TFT TTT TFT FFF TFT TTT TFT TTT FFF FTF FFF FTF TTT FTF FFF FTF FFF
\___________________________________/ \___________________________________/
"standard" Menger sponge complement
alternates the "standard" Menger sponge and its complement.
Obviously many other rules do exist as shown below...
0 --> F
1 --> T
(Please note that the preceding cube numbers include the full cubes as well as the empty/missing ones)
110 010 010 000 111 111 011 010 101
000 100 010 000 101 101 000 110 000
100 011 010 011 000 100 110 001 100
110 001 010 001 011 100 000 001 101