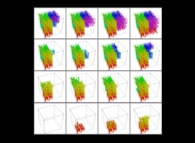
Bidimensional Hilbert Curve -iteration 5- [Courbe de Hilbert bidimensionnelle -itération 5-].

See various bidimensional Hilbert and Peano curves (possibly including this one):

See bidimensional Hilbert curves, their nodes being "loaded" with some data (related to prime numbers, real number digits,...):

See the used color set to display the parameter T.

See the used color set to display the pi digits.

See various tridimensional Hilbert and Peano curves (possibly including this one):

See tridimensional Hilbert curves, their nodes being "loaded" with some data (related to prime numbers, real number digits,...):

See the used color set to display the parameter T.

See the used color set to display the pi digits.

The bidimensional Peano Surjection:

Giuseppe Peano defined the following surjection :

                    [0,1] --> [0,1]x[0,1]

Let's T being a real number defined using the base 3 :

                    T    = 0.A₁A₂A₃... ∈ [0,1] with A_i ∈ {0,1,2}

Let's X(T) and Y(T) being two real functions of T defined as :

                    X(T) = 0.B₁B₂B₃... ∈ [0,1] with B_i ∈ {0,1,2}

                    Y(T) = 0.C₁C₂C₃... ∈ [0,1] with C_i ∈ {0,1,2}

with :

                    B_n = A_2n-1 if A₂+A₄+...+A_2n-0 is even

                    B_n = 2-A_2n-1 otherwise

                    C_n = A_2n if A₁+A₃+...+A_2n-1 is even

                    C_n = 2-A_2n otherwise

These two functions X(T) and Y(T) are the coordinates of a point P(T) inside the [0,1]x[0,1] square. The displayed "curve" -as little spheres- is the trajectory of P(T) when T varies from 0 (lower left corner) to 1-epsilon (upper right corner).

Here are the four first bidimensional Peano curves with an increasing number of digits {2,4,6,8}:

[See the used color set to display the parameter T]

2-The tridimensional Peano Surjection:

A tridimensional surjection can be defined :

                    [0,1] --> [0,1]x[0,1]x[0,1]

as a generalization of the bidimensional one.

Let's T being a real number defined using the base 3 :

                    T    = 0.A₁A₂A₃... ∈ [0,1] with A_i ∈ {0,1,2}

Let's X(T), Y(T) and Z(T) being three real functions of T defined as:

                    X(T) = 0.B₁B₂B₃... ∈ [0,1] with B_i ∈ {0,1,2}

                    Y(T) = 0.C₁C₂C₃... ∈ [0,1] with C_i ∈ {0,1,2}

                    Y(T) = 0.D₁D₂D₃... ∈ [0,1] with D_i ∈ {0,1,2}

with :

                    B_n = A_3n-2 if A₃+A₆+...+A_3n-0 is even

                    B_n = 2-A_3n-2 otherwise

                    C_n = A_3n-1 if A₂+A₅+...+A_3n-1 is even

                    C_n = 2-A_3n-1 otherwise

                    D_n = A_3n if A₁+A₄+...+A_3n-2 is even

                    D_n = 2-A_3n otherwise

These three functions X(T), Y(T) and Z(T) are the coordinates of a point P(T) inside the [0,1]x[0,1]x[0,1] cube. The displayed "curve" is the trajectory of P(T) -displayed as little spheres- when T varies from 0 (lower left corner) to 1-epsilon (upper right corner).

Here are the three first tridimensional Peano curves with an increasing number of digits {3,6,9}:

[See the used color set to display the parameter T]

The bidimensional Hilbert Curves:

Let's C₁(T) being a parametric curve

defined by means of 2 real functions of T (T ∈ [0,1]) X₁(T) ∈ [0,1] and Y₁(T) ∈ [0,1] such as :

                    X₁(T=0)=0 Y₁(T=0)=0 (lower left corner)

                    X₁(T=1)=1 Y₁(T=1)=0 (lower right corner)

Then one defines a sequence of curves C_i(T) (i >= 1) as follows :

                    C_i(T) = {X_i(T),Y_i(T)} ∈ [0,1]x[0,1] --> C_i+1(T) = {X_i+1(T),Y_i+1(T)} ∈ [0,1]x[0,1]

                    if T ∈ [0,1/4[:
                              X_i+1(T) =   Y_i(4T-0)
                              Y_i+1(T) =   X_i(4T-0)
                                                   Transformation 1

                    if T ∈ [1/4,2/4[:
                              X_i+1(T) =   X_i(4T-1)
                              Y_i+1(T) = 1+Y_i(4T-1)
                                                   Transformation 2

                    if T ∈ [2/4,3/4[:
                              X_i+1(T) = 1+X_i(4T-2)
                              Y_i+1(T) = 1+Y_i(4T-2)
                                                   Transformation 3

                    if T ∈ [3/4,1]:
                              X_i+1(T) = 2-Y_i(4T-3)
                              Y_i+1(T) = 1-X_i(4T-3)
                                                   Transformation 4

Please note that 4=2^d where d=2 is the space dimension.

See a special C₁(T) curve

in order to understand the geometrical meaning of the 4 transformations

and of their order

.

Here are the five first bidimensional Hilbert curves with an increasing number of iterations :

[See the used color set to display the parameter T]

Here are some examples of Hilbert-like bidimensional curves using different generating curves :

The tridimensional Hilbert Curves:

Let's C₁(T) being a parametric curve

defined by means of 3 real functions of T (T ∈ [0,1]) X₁(T) ∈ [0,1], Y₁(T) ∈ [0,1] and Z₁(T) ∈ [0,1] such as :

                    X₁(T=0)=0 Y₁(T=0)=0 Z₁(T=0)=0 (lower left foreground corner)

                    X₁(T=1)=1 Y₁(T=1)=0 Z₁(T=1)=0 (lower right foreground corner)

Then one defines a sequence of curves C_i(T) (i >= 1) as follows :

                    C_i(T) = {X_i(T),Y_i(T),Z_i(T)} ∈ [0,1]x[0,1]x[0,1] --> C_i+1(T) = {X_i+1(T),Y_i+1(T),Z_i+1(T)} ∈ [0,1]x[0,1]x[0,1]

                    if T ∈ [0,1/8[:
                              X_i+1(T) =   X_i(8T-0)
                              Y_i+1(T) =   Z_i(8T-0)
                              Z_i+1(T) =   Y_i(8T-0)
                                                   Transformation 1

                    if T ∈ [1/8,2/8[:
                              X_i+1(T) =   Z_i(8T-1)
                              Y_i+1(T) = 1+Y_i(8T-1)
                              Z_i+1(T) =   X_i(8T-1)
                                                   Transformation 2

                    if T ∈ [2/8,3/8[:
                              X_i+1(T) = 1+X_i(8T-2)
                              Y_i+1(T) = 1+Y_i(8T-2)
                              Z_i+1(T) =   Z_i(8T-2)
                                                   Transformation 3

                    if T ∈ [3/8,4/8[:
                              X_i+1(T) = 1+Z_i(8T-3)
                              Y_i+1(T) = 1-X_i(8T-3)
                              Z_i+1(T) = 1-Y_i(8T-3)
                                                   Transformation 4

                    if T ∈ [4/8,5/8[:
                              X_i+1(T) = 2-Z_i(8T-4)
                              Y_i+1(T) = 1-X_i(8T-4)
                              Z_i+1(T) = 1+Y_i(8T-4)
                                                   Transformation 5

                    if T ∈ [5/8,6/8[:
                              X_i+1(T) = 1+X_i(8T-5)
                              Y_i+1(T) = 1+Y_i(8T-5)
                              Z_i+1(T) = 1+Z_i(8T-5)
                                                   Transformation 6

                    if T ∈ [6/8,7/8[:
                              X_i+1(T) = 1-Z_i(8T-6)
                              Y_i+1(T) = 1+Y_i(8T-6)
                              Z_i+1(T) = 2-X_i(8T-6)
                                                   Transformation 7

                    if T ∈ [7/8,1]:
                              X_i+1(T) =   X_i(8T-7)
                              Y_i+1(T) = 1-Z_i(8T-7)
                              Z_i+1(T) = 2-Y_i(8T-7)
                                                   Transformation 8

Please note that 8=2^d where d=3 is the space dimension.

See a special C₁(T) curve

in order to understand the geometrical meaning of the 8 transformations

and of their order

.

Here are the four first tridimensional Hilbert curves with an increasing number of iterations :

[See the used color set to display the parameter T]

Here are some examples of Hilbert-like tridimensional curves using different generating curves and in particular "open" knots :