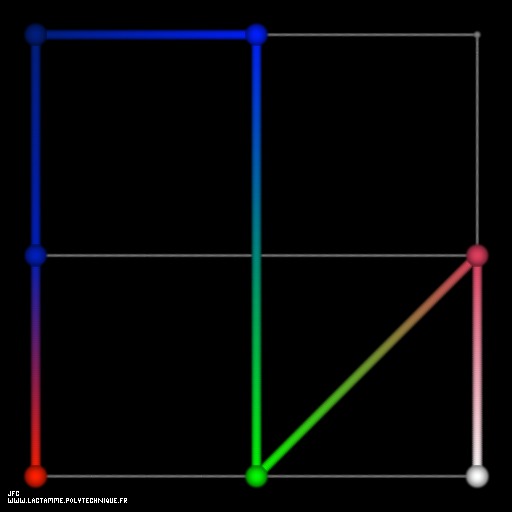
AVirtualMachineForExploringSpaceTimeAndBeyond : Bidimensional display of 7 Rational Numbers by means of the Stern-Brocot Tree (Visualisation bidimensionnelle de 7 Nombre Rationnels a l'aide de l'arbre de Stern-Brocot).

Bidimensional display of 7 Rational Numbers by means of the Stern-Brocot Tree [Visualisation bidimensionnelle de 7 Nombre Rationnels à l'aide de l'arbre de Stern-Brocot].

Let's define the so-called Median-Mean of two rational numbers A/B and C/D:

                                           A+C
                    MedianMean(A/B,C/D) = -----
                                           B+D

Nota: the Median-Mean is at the origin of the so-called Simpson Paradox.

'Left', 'Right' and 'Mean' being three rational numbers, the following recursive algorithm:

                    Generate(Left,Right)
                         {
                         Mean = MedianMean(Left,Right);
                          
                         Generate(Left,Mean);
                         Generate(Mean,Right);
                         }

starting with:

                    zero=0/1
                    infinity=1/0
                     
                    Generate(zero,infinity)

gives the Stern-Brocot tree where all the positive rational numbers (Q⁺={Mean}) appear once and only once:

                    
                    level=1   0/1 > > > > > > > > > > > > > > > > > > 1/1 < < < < < < < < < < < < < < < < < < 1/0
                               |                                     * | *                                     |
                               |                                   *   |   *                                   |
                               |                                 *     |     *                                 |
                               |                               *       |       *                               |
                               |                             *         |         *                             |
                               |                           *           |           *                           |
                               |                         *             |             *                         |
                               |                       *               |               *                       |
                               |                     *                 |                 *                     |
                    level=2   0/1 > > > > > > > > 1/2 < < < < < < < < 1/1 > > > > > > > > 2/1 < < < < < < < < 1/0
                               |                 * | *                 |                 * | *                 |
                               |               *   |   *               |               *   |   *               |
                               |             *     |     *             |             *     |     *             |
                               |           *       |       *           |           *       |       *           |
                    level=3   0/1 > > > 1/3 < < < 1/2 > > > 2/3 < < < 1/1 > > > 3/2 < < < 2/1 > > > 3/1 < < < 1/0
                               |       * | *     * | *     * | *     * | *     * | *     * | *     * | *       |
                               |     *   |   * *   |   * *   |   * *   |   * *   |   * *   |   * *   |   *     |
                             (...)     (...)     (...)     (...)     (...)     (...)     (...)     (...)     (...)

At last, the colored points display the Rational Numbers {1/1,1/2,1/3,2/3,2/1,3/2,3/1} with coordinates {X=numerator,Y=denominator} and a luminance proportional to their decimal values.

See some related pictures (including this one):