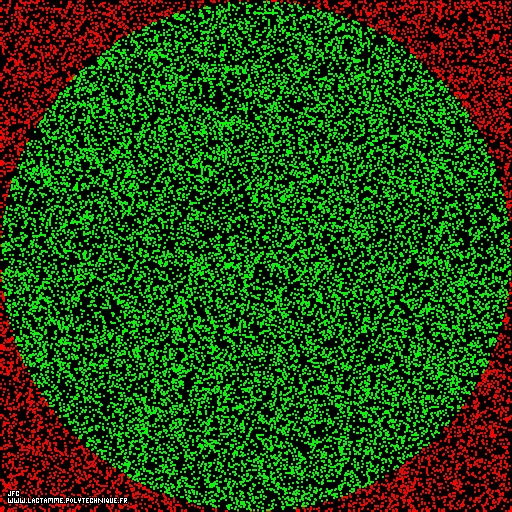
How to compute 'pi' with a gun [Comment calculer 'pi' avec un fusil].
It suffices to use a square target with an inscribed circle inside.
Then shoot randomly the target.
In this picture there are NG=20692 shots inside the circle (Green points) and NR=5719 outside (Red points).
The full square contains NG+NR=20692+5719=26411 points (Green and Red).
Please note that two shots fired at the same point count as one and only one.
R being the circle radius, the areas of the circle and the square are respectively:
2
C = pi*R
2
S = (2*R)
K being a certain constant, C and S can be approximated using the point numbers:
C ~ K*NG
S ~ K*(NG+NR)
Hence:
2
C pi*R pi
--- = -------- = ----
S 2 4
(2*R)
C K*NG 20692
pi = 4*--- ~ 4*----------- = 4*------- = 3.13
S K*(NG+NR) 26411
assuming a perfect random process...
(CMAP28 WWW site: this page was created on 09/08/2021 and last updated on 02/25/2024 11:31:24 -CET-)
[See the generator of this picture [Voir le générateur de cette image]]
[See all related pictures (including this one) [Voir toutes les images associées (incluant celle-ci)]]
[Please visit the related ImagesDidactiques picture gallery [Visitez la galerie d'images ImagesDidactiques associée]]
[Please visit the related NumberTheory picture gallery [Visitez la galerie d'images NumberTheory associée]]
[Go back to AVirtualMachineForExploringSpaceTimeAndBeyond [Retour à AVirtualMachineForExploringSpaceTimeAndBeyond]]
[The Y2K Bug [Le bug de l'an 2000]]
[Site Map, Help and Search [Plan du Site, Aide et Recherche]]
[Mail [Courrier]]
[About Pictures and Animations [A Propos des Images et des Animations]]
Copyright © Jean-François COLONNA, 2021-2024.
Copyright © CMAP (Centre de Mathématiques APpliquées) UMR CNRS 7641 / École polytechnique, Institut Polytechnique de Paris, 2021-2024.

