The abelian -commutative- group defined on elliptic curves [Le groupe abélien -commutatif- défini sur les courbes elliptiques].
[See the so-called last Fermat's theorem]
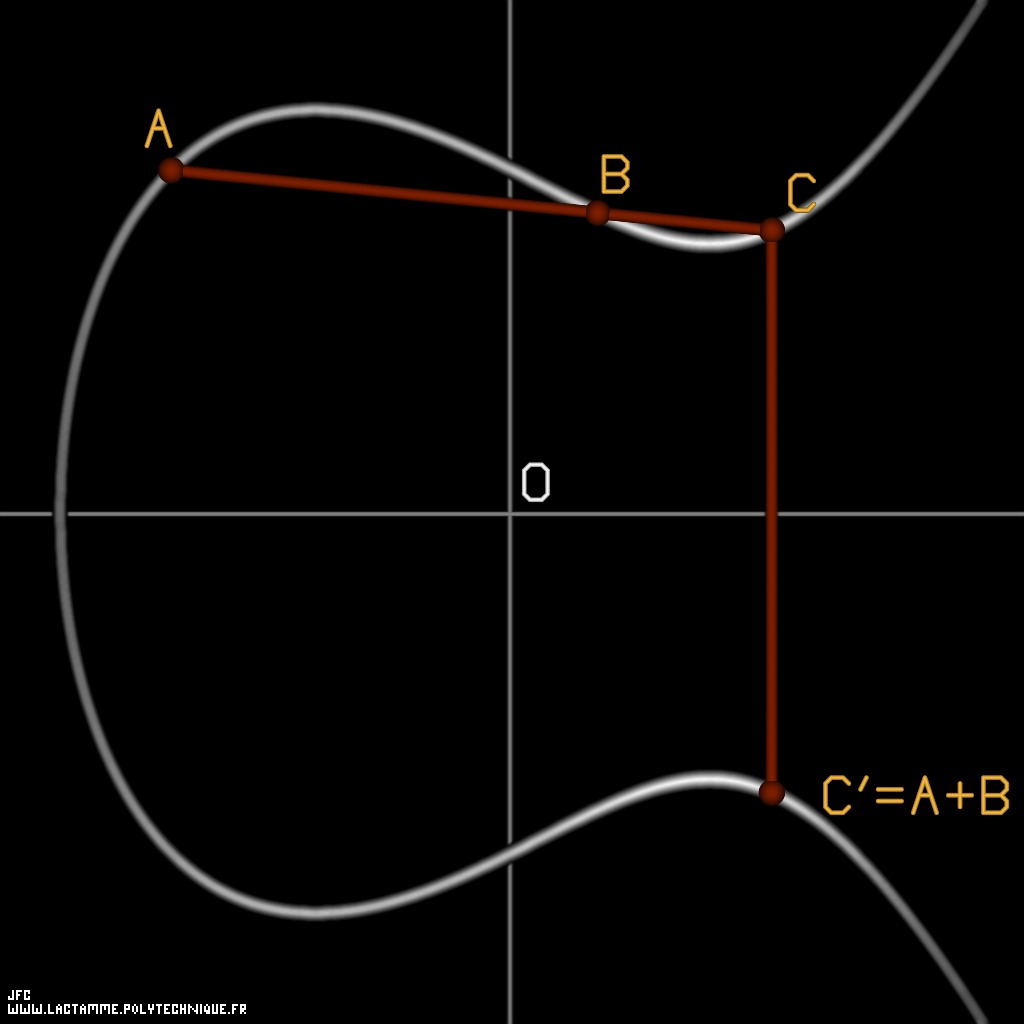
The continuous white line displays the following elliptic curve:
y2 = x3 - x + 1
An elliptic curve is an abelian -i.e. commutative- group:
- Definitions:
- I (the identity of the group) is the point at infinity (a vertical line on the picture).
- The inverse of a point P=P(x,y) is -P=P(x,-y). On the picture R' means -R and defines P+Q.
- P+Q+R = I
- Abelian group laws:
- P+I = I+P = P [Identity]
- P+(-P) = (-P)+P = I [Inverse]
- (P+Q)+R = P+(Q+R) [Associativity]
- P+Q = Q+P [Commutativity]
On this picture, the 3 points P, Q and R have rational coordinates:
1 1
P = {- --- , + ---}
1 1
1 7
Q = {+ --- , + ---}
4 8
19 103
R = {+ ---- , + -----}
25 125
(CMAP28 WWW site: this page was created on 09/18/2013 and last updated on 01/20/2025 11:23:51 -CET-)
[See the generator of this picture [Voir le générateur de cette image]]
[See all related pictures (including this one) [Voir toutes les images associées (incluant celle-ci)]]
[Please visit the related ImagesDidactiques picture gallery [Visitez la galerie d'images ImagesDidactiques associée]]
[Please visit the related NumberTheory picture gallery [Visitez la galerie d'images NumberTheory associée]]
[Go back to AVirtualMachineForExploringSpaceTimeAndBeyond [Retour à AVirtualMachineForExploringSpaceTimeAndBeyond]]
[The Y2K Bug [Le bug de l'an 2000]]
[Site Map, Help and Search [Plan du Site, Aide et Recherche]]
[Mail [Courrier]]
[About Pictures and Animations [A Propos des Images et des Animations]]
Copyright © Jean-François COLONNA, 2013-2025.
Copyright © CMAP (Centre de Mathématiques APpliquées) UMR CNRS 7641 / École polytechnique, Institut Polytechnique de Paris, 2013-2025.

