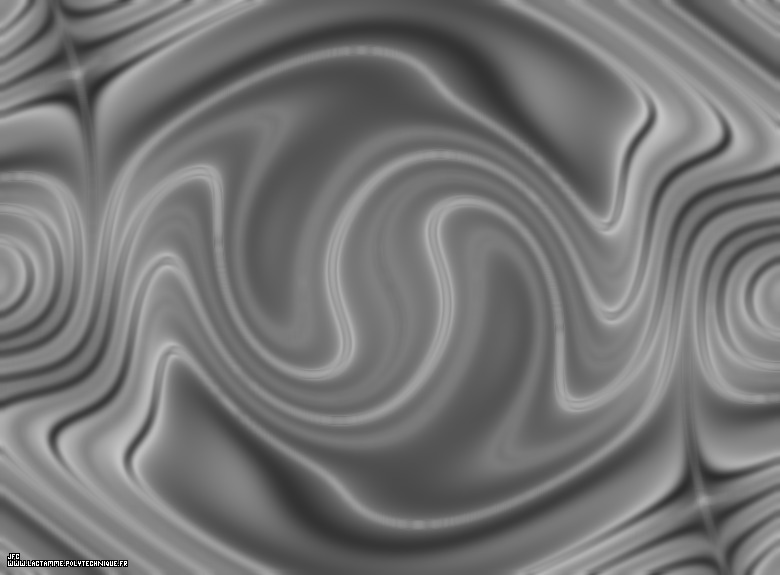
Bidimensional display of the integration of the Lorenz attractor system for 448500 different initial conditions [Visualisation bidimensionnelle de l'intégration du système de l'attracteur de Lorenz pour 448500 conditions initiales différentes].

Bidimensional display of the integration of the Lorenz attractor system for 448500 different initial conditions [Visualisation bidimensionnelle de l'intégration du système de l'attracteur de Lorenz pour 448500 conditions initiales différentes].
x = X scaled within [-30.0,+30.0],
0
y = Y scaled within [-30.0,+30.0],
0
z = GREY(X,Y) within [0.0,+60.0],
0
where 'GREY' denotes a function of the grey level of the
current point. This picture  displays
the 448500 integrations during 255 time steps; the initial conditions can
be viewed as the lower left picture and are computed as a gaussian field.
For each frame of this animation, the results are encoded as follows:
displays
the 448500 integrations during 255 time steps; the initial conditions can
be viewed as the lower left picture and are computed as a gaussian field.
For each frame of this animation, the results are encoded as follows:
X = x (unchanged),
0
Y = y (unchanged),
0
GREY(X,Y) = z
a black and white scale is used -white points are the
closest ones when black ones are the farthest-.
Finally, this picture  and this one
and this one  exhibit
a tridimensional rendering.
exhibit
a tridimensional rendering.
 and
tridimensionally
and
tridimensionally  displayed respectively during 127 time steps (the lower
left picture exhibits the initial conditions).
displayed respectively during 127 time steps (the lower
left picture exhibits the initial conditions).