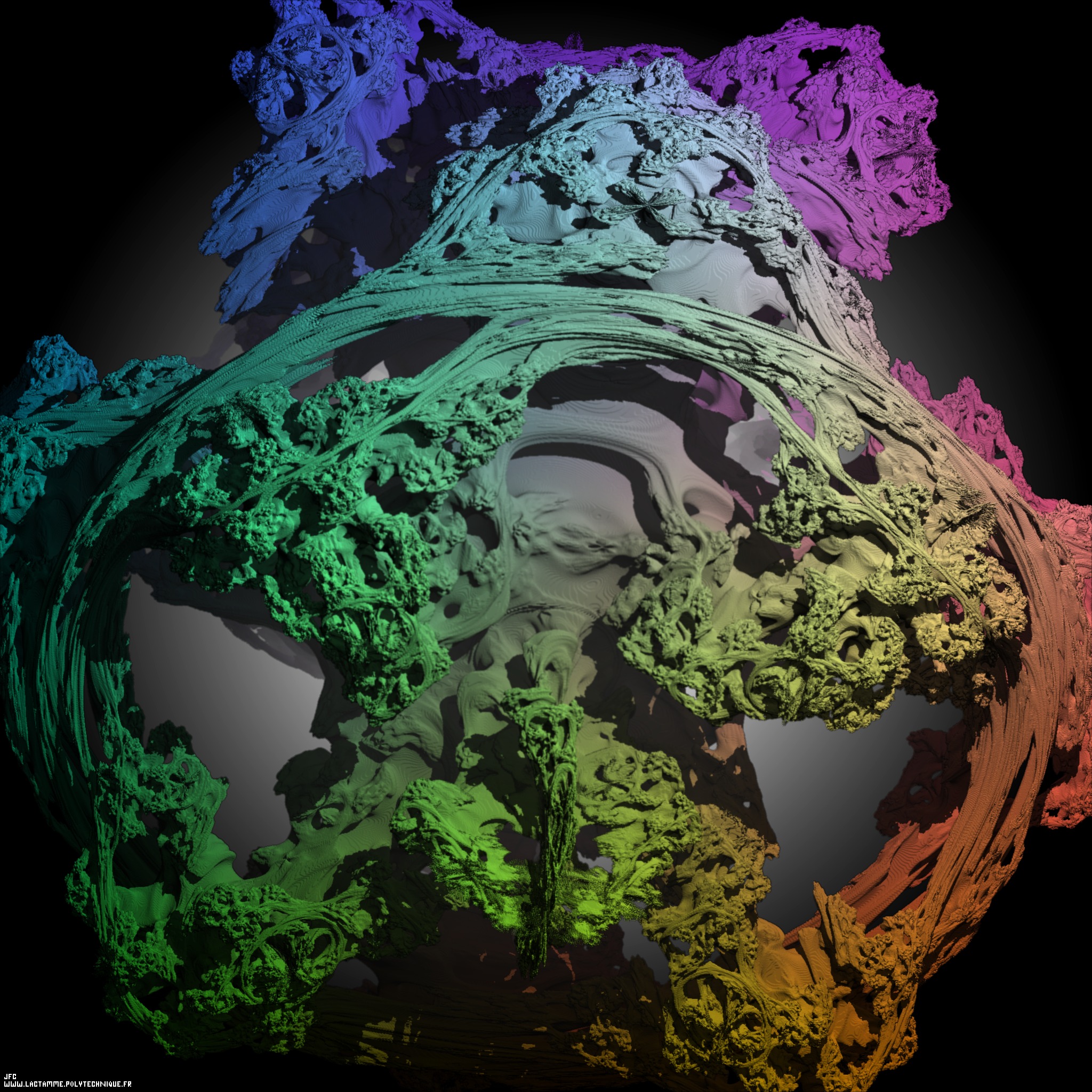
A foggy pseudo-octonionic Julia set ('MandelBulb' like: a 'JuliaBulb') computed with A=(-0.581514...,+0.635888...,0,0,0,0,0,0) and with a rotation about the X axis -tridimensional cross-section- [Un ensemble de Julia brumeux dans l'ensemble des pseudo-octonions (comme un 'MandelBulb': un 'JuliaBulb') calculé pour A=(-0.581514...,+0.635888...,0,0,0,0,0,0) et avec une rotation autour de l'axe X -section tridimensionnelle-].
P(o) = 1*o + {-0.5815147625160462,+0.6358885017421603,0,0,0,0,0,0}
4
fR(R ,R ) = (R *R )
1 2 1 2
fA1(A1 ,A1 ) = 4*(A1 +A1 )
1 2 1 2
fA2(A2 ,A2 ) = 4*(A2 +A2 )
1 2 1 2
fA3(A3 ,A3 ) = 4*(A3 +A3 )
1 2 1 2
fA4(A4 ,A4 ) = 4*(A4 +A4 )
1 2 1 2
fA5(A5 ,A5 ) = 4*(A5 +A5 )
1 2 1 2
fA6(A6 ,A6 ) = 4*(A6 +A6 )
1 2 1 2
fA7(A7 ,A7 ) = 4*(A7 +A7 )
1 2 1 2
