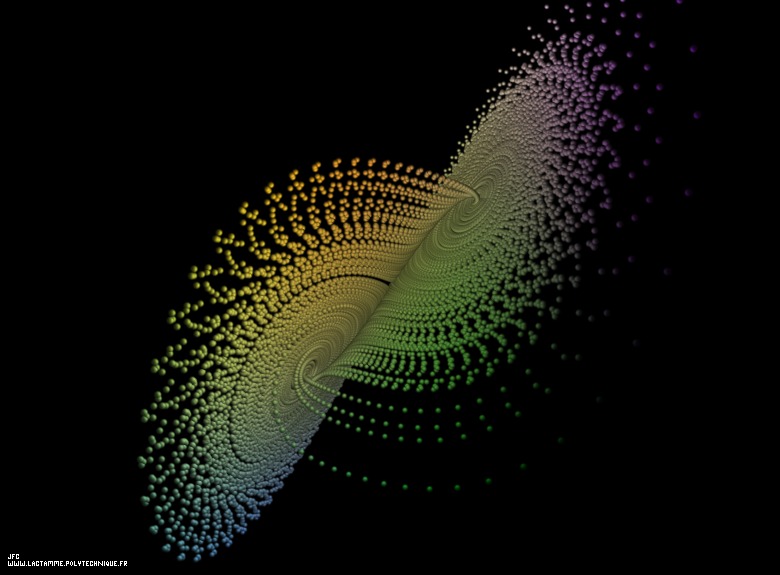
The Lorenz attractor [L'attracteur de Lorenz].

The Lorenz attractor [L'attracteur de Lorenz].
 See its rotation.
See its rotation.
 See a set of 4x3 stereograms.
See a set of 4x3 stereograms.
- dx
| ---- = -10x + 10y
| dt
|
| dy
< ---- = 28x - y - xz
| dt
|
| dz 8
| ---- = - ---z + xy
- dt 3
This picture is obtained integrating these equations using the Runge-Kutta
method of the fourth order with:
{X ,Y ,Z } = {0.01,0.01,0.01}
0 0 0
Dt = 0.01
each sphere displays a time step and its attributes are chosen as follows:
RADIUS = constant
RED = K.Dx
GREEN = K.Dy
BLUE = K.Dz
where K denotes a renormalization factor and {Dx,Dy,Dz} are the results of
the numerical integration process.
 sensitivity to initial conditions,
the
sensitivity to initial conditions,
the  sensitivity to integration methods
and the
sensitivity to integration methods
and the  sensitivity to rounding-off errors.
sensitivity to rounding-off errors.
- dx
| ---- = -10x + 10y
| dt
|
| dy
< ---- = 28x - y - xz
| dt
|
| dz 8
| ---- = - ---z + xy
- dt 3
Cette image présente la trajectoire que décrit le
système au cours du temps dans l'espace
[x,y,z], à partir d'une condition initiale arbitraire ({0.01,0.01,0.01}).
La couleur n'a pas ici qu'une valeur artistique;
elle véhicule une information pertinente: les
intensités des trois couleurs fondamentales
(le Rouge, le Vert et le Bleu) sont
proportionnelles respectivement aux trois
dérivées en t définies ci-dessus.

